Now that you think you’ve solved our brainteasers, it’s time to check your answers. Maybe you got them all correct. Maybe you were stumped on one of them…but surely not all of them. Whatever the case may be,
we know you enjoyed taking your mental break.
1.) Fence Me In!
The puzzle factory has twenty robots on the factory floor, laid out as shown on a grid. The safety manager wants to install fencing on the plant floor to isolate the robots into separate work cells. The plant manager agrees, but only if it can be done with six (and only six) straight sections of fence. Can you determine where the six straight fence sections should be placed?
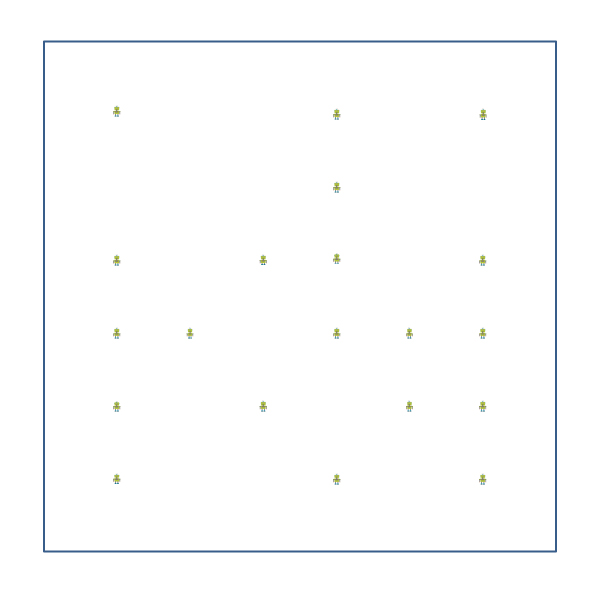
Answer:

[hozbreak]
2.) Can’t Touch This
Can you position six Edison fuses so that each one is touching all of the other five? We’re told it can even be done with seven (with each touching the other 6, of course)?
Answer:


[hozbreak]
3.) Month of Sundays
There have been a number of (false) rumors going around the internet about how rare it is for a certain month to have 5 weekends. The time frame of 823 years is often quoted, and you will purportedly get rich that month IF you forward the email to 5 or 10 of your friends. That last part is a dead-give-a-way that something is rotten in Denmark, right? Five-weekend-months happen pretty often – April (2017) will be one (and with only 30 days in that month).
If you want to include 5 Fridays with those 5 weekends, you only have to wait until this December (2017).
On a related note, we’ve seen questions and posts asking about years that have Februarys with five Wednesdays (or any other day of the week, for that matter). Obviously, that requires the year in question to be a leap year, and
February 1st of that year must fall on a Wednesday (or your favorite day-of-the-week). One question that is often posed, “Find the probability that the month of February may have 5 Wednesdays in A) a leap year, and B) a non-leap year.” Not a bad question, but not really a difficult problem to solve either. Let’s make it more of a challenge: What is the probability that ANY given year will have a February with five Wednesdays? (Remember that leap years don’t fall EVERY four years – just mostly every four years.

Answer: 24.25% / 7 = 3.464%
Assuming you are starting in the year 1582 and using the Gregorian calendar, then the probability that ANY YEAR is a Leap Year is 24.25%
In the Gregorian calendar, there is one leap year every four years, with a correction that skips three leap days every four hundred years (it skips years that are divisible by 100 but not divisible by 400, so the years 2000 and 2400 are leap years but 2100, 2200, and 2300 will not be). So, in any 400 year period, there are 97 leap years (100 years divisible by 4 less those that end in 00 but are not divisible by 400). So the probability is 97/400, or 0.2425
And the probability that such a year has a Wednesday, February 1st is one in seven. So therefor: the probability that any given year would have a February with 5 Wednesdays is 24.25% / 7 = 3.464%
[hozbreak]